We will be concerned primarily with bijective proofs, i.e., showing that two sets have the same number of elements by exhibiting a bijection (one-to-one correspondence) between them.
我们主要关心的是对射的
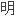 ,也就是透过在两个集合间建立一个对射(一对一
,也就是透过在两个集合间建立一个对射(一对一
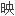
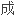 的
的 数)
数)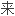

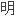 它们的元素个数相等。
它们的元素个数相等。
We will be concerned primarily with bijective proofs, i.e., showing that two sets have the same number of elements by exhibiting a bijection (one-to-one correspondence) between them.
我们主要关心的是对射的
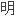 ,也就是透过在两个集合间建立一个对射(一对一
,也就是透过在两个集合间建立一个对射(一对一
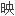
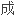 的
的 数)
数)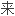

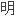 它们的元素个数相等。
它们的元素个数相等。
声 :以上例句、词性分类均由互联网资源自动生
:以上例句、词性分类均由互联网资源自动生 ,部分未经过人工审核,其表达内容亦不代表本软件的观点;若发现问题,欢迎向我们指正。
,部分未经过人工审核,其表达内容亦不代表本软件的观点;若发现问题,欢迎向我们指正。
We will be concerned primarily with bijective proofs, i.e., showing that two sets have the same number of elements by exhibiting a bijection (one-to-one correspondence) between them.
我们主要关心的是对射的证明,也就是透过在两 集合间建
集合间建

 对射(
对射( 对
对 且映成的
且映成的
 )来证明它们的元素
)来证明它们的元素

 等。
等。
声明:以上例句、词性分类均由互联网资源自动生成,部分未经过人工审核,其表达内容亦不代表本软件的观点;若发现问题,欢迎向我们指正。
We will be concerned primarily with bijective proofs, i.e., showing that two sets have the same number of elements by exhibiting a bijection (one-to-one correspondence) between them.
我们主要关心的
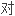
 的证明,也就
的证明,也就 透过在两个集合间建立一个
透过在两个集合间建立一个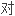
 (一
(一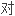 一且映成的
一且映成的 数)来证明它们的元素个数相等。
数)来证明它们的元素个数相等。
声明:以上例句、词性分类均由互联
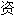
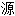 自动生成,部分未经过人工审核,其表达内容亦不代表本软件的观点;若发现问题,欢迎向我们指正。
自动生成,部分未经过人工审核,其表达内容亦不代表本软件的观点;若发现问题,欢迎向我们指正。
We will be concerned primarily with bijective proofs, i.e., showing that two sets have the same number of elements by exhibiting a bijection (one-to-one correspondence) between them.
我们主要关心 是对射
是对射
 明,也就是透过在两个集合间建立一个对射(一对一且
明,也就是透过在两个集合间建立一个对射(一对一且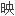
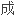


 )
)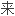
 明它们
明它们 元素个
元素个 相等。
相等。
声明:以上例句、词性分类均由互联网资源自动生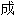 ,部分未经过人工审核,其表达内容亦不代表本软件
,部分未经过人工审核,其表达内容亦不代表本软件 观点;若发现问题,欢迎向我们指正。
观点;若发现问题,欢迎向我们指正。
We will be concerned primarily with bijective proofs, i.e., showing that two sets have the same number of elements by exhibiting a bijection (one-to-one correspondence) between them.
我们主要关心的是对射的证明,也就是透过在两个集合间建立一个对射(一对一且映成的 数)来证明它们的元素个数相等。
数)来证明它们的元素个数相等。
声明:以上例句、词性分类均由互联网资源自动生成,部分未经过人工审核,其表达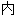

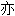 不代表本软件的观点;若发现问题,欢迎向我们指正。
不代表本软件的观点;若发现问题,欢迎向我们指正。
We will be concerned primarily with bijective proofs, i.e., showing that two sets have the same number of elements by exhibiting a bijection (one-to-one correspondence) between them.
我们主要关心

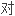 射
射 证明,也就
证明,也就 透过在两个集合间建立一个
透过在两个集合间建立一个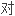 射(一
射(一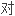 一且映成
一且映成
 数)来证明它们
数)来证明它们 元素个数相等。
元素个数相等。
声明:以上例句、词性分类均由互联网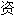
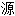
 动生成,部分未经过人工审核,其表达内容亦不代表本软件
动生成,部分未经过人工审核,其表达内容亦不代表本软件 观点;若发现问题,欢迎向我们指正。
观点;若发现问题,欢迎向我们指正。
We will be concerned primarily with bijective proofs, i.e., showing that two sets have the same number of elements by exhibiting a bijection (one-to-one correspondence) between them.
我们主要关心的是对射的证明,也就是透过在两个集合间

 个对射(
个对射( 对
对 且映成的
且映成的
 )来证明它们的元素个
)来证明它们的元素个

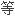 。
。
声明:以上例句、词性分类均由互联网资源自动生成,部分未经过人工审核,其表达内容亦不代表本软件的观点;若发现问题,欢迎向我们指正。
We will be concerned primarily with bijective proofs, i.e., showing that two sets have the same number of elements by exhibiting a bijection (one-to-one correspondence) between them.
我们主要关心的是对射的证明,也就是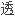
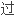
 两个集合间建立一个对射(一对一且映成的
两个集合间建立一个对射(一对一且映成的 数)来证明它们的元素个数相等。
数)来证明它们的元素个数相等。
声明:以上例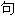 、词
、词 分类均由互联网资源自动生成,部分未经
分类均由互联网资源自动生成,部分未经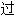 人工审核,其表达内容亦不代表本软件的观点;若发现问题,欢迎向我们指正。
人工审核,其表达内容亦不代表本软件的观点;若发现问题,欢迎向我们指正。
We will be concerned primarily with bijective proofs, i.e., showing that two sets have the same number of elements by exhibiting a bijection (one-to-one correspondence) between them.
我们主要关心的是对射的证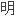 ,也就是透过在两个
,也就是透过在两个

 建立一个对射(一对一且映成的
建立一个对射(一对一且映成的 数)来证
数)来证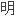 它们的元素个数相等。
它们的元素个数相等。

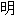 :
: 上例句、词性分类均由互联网资源自动生成,部分未经过人工审核,其表达内容亦不代表本软件的观点;若发现问题,欢迎向我们指正。
上例句、词性分类均由互联网资源自动生成,部分未经过人工审核,其表达内容亦不代表本软件的观点;若发现问题,欢迎向我们指正。