A series of the necessary and Sufficient conditions of the diagonalizable orthogonaltransformation and orthogonal matrix is given.
获得 一种求可对角化正
一种求可对角化正 矩阵的特征向
矩阵的特征向 的简便方法。
的简便方法。
A series of the necessary and Sufficient conditions of the diagonalizable orthogonaltransformation and orthogonal matrix is given.
获得 一种求可对角化正
一种求可对角化正 矩阵的特征向
矩阵的特征向 的简便方法。
的简便方法。
One kind of inverse eigenvalue problems, whose solutions are required to be normal or diagonalizable matrices, is investigated in quaternionic quantum mechanics.
摘要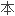

 究
究 四元数
四元数 子力学中一类要求其解是正规或可对角化四元数矩阵的特征值反问题。
子力学中一类要求其解是正规或可对角化四元数矩阵的特征值反问题。
This paper discusses the structure, calculation of multiplication and power, eigenvalue and eigenvector, and diagonalizable problems of matrix of rank equal to 1.
摘要对秩等于1的矩阵的结构、乘法与乘幂运算、特征值与特征向 和对角化问题进行
和对角化问题进行 讨论。
讨论。
声明:以上例句、词性分类均由互联网资源自动生成,部分未经过人工审核,其表达内容亦不代表 软件的观点;若发现问题,欢迎向我们指正。
软件的观点;若发现问题,欢迎向我们指正。

 角化
角化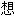 词
词A series of the necessary and Sufficient conditions of the diagonalizable orthogonaltransformation and orthogonal matrix is given.
获得 一种求
一种求
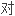 角化正文矩阵的特征向量的简便方法。
角化正文矩阵的特征向量的简便方法。
One kind of inverse eigenvalue problems, whose solutions are required to be normal or diagonalizable matrices, is investigated in quaternionic quantum mechanics.
摘要本文研究 四元数量子力学中一类要求其解是正规
四元数量子力学中一类要求其解是正规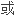

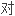 角化四元数矩阵的特征值反问题。
角化四元数矩阵的特征值反问题。
This paper discusses the structure, calculation of multiplication and power, eigenvalue and eigenvector, and diagonalizable problems of matrix of rank equal to 1.
摘要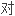 秩等于1的矩阵的结构、乘法与乘幂运算、特征值与特征向量和
秩等于1的矩阵的结构、乘法与乘幂运算、特征值与特征向量和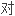 角化问题进行
角化问题进行 讨论。
讨论。
声明:以上例句、词性分类均由互联网资源自动生成,部分未经过人工审核,其表达内容亦不代表本软件的观点;若发现问题,欢迎向我们指正。
A series of the necessary and Sufficient conditions of the diagonalizable orthogonaltransformation and orthogonal matrix is given.
获得 一种求可对角化正文矩阵
一种求可对角化正文矩阵 特征向量
特征向量

 方法。
方法。
One kind of inverse eigenvalue problems, whose solutions are required to be normal or diagonalizable matrices, is investigated in quaternionic quantum mechanics.
摘要本文研究 四元数量子力学中一类要求其解是正规或可对角化四元数矩阵
四元数量子力学中一类要求其解是正规或可对角化四元数矩阵 特征值反问题。
特征值反问题。
This paper discusses the structure, calculation of multiplication and power, eigenvalue and eigenvector, and diagonalizable problems of matrix of rank equal to 1.
摘要对秩等于1 矩阵
矩阵 结构、乘法与乘幂运算、特征值与特征向量和对角化问题进行
结构、乘法与乘幂运算、特征值与特征向量和对角化问题进行 讨论。
讨论。
声明:以上例句、词性分类均由互联网资源自动生成,部分未经过人工审核,其表达内容亦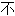 代表本软件
代表本软件 观点;若发现问题,欢迎向我们指正。
观点;若发现问题,欢迎向我们指正。
A series of the necessary and Sufficient conditions of the diagonalizable orthogonaltransformation and orthogonal matrix is given.
获得 一种求可对角化正文矩
一种求可对角化正文矩

 征向量
征向量 简便方法。
简便方法。
One kind of inverse eigenvalue problems, whose solutions are required to be normal or diagonalizable matrices, is investigated in quaternionic quantum mechanics.
摘要本文研究 四元数量子力学中一类要求其解是正规或可对角化四元数矩
四元数量子力学中一类要求其解是正规或可对角化四元数矩

 征值反问题。
征值反问题。
This paper discusses the structure, calculation of multiplication and power, eigenvalue and eigenvector, and diagonalizable problems of matrix of rank equal to 1.
摘要对秩等于1 矩
矩
 结构、乘法与乘幂运算、
结构、乘法与乘幂运算、 征值与
征值与 征向量和对角化问题进行
征向量和对角化问题进行 讨论。
讨论。
声明:以上例句、词 分类均由互联网资源自动生成,部分未经过人工审核,其表达内容亦不代表本软件
分类均由互联网资源自动生成,部分未经过人工审核,其表达内容亦不代表本软件 观点;若发现问题,欢迎向我们指正。
观点;若发现问题,欢迎向我们指正。
A series of the necessary and Sufficient conditions of the diagonalizable orthogonaltransformation and orthogonal matrix is given.
获得 一种求可对角化正文矩阵
一种求可对角化正文矩阵 特征向量
特征向量

 方法。
方法。
One kind of inverse eigenvalue problems, whose solutions are required to be normal or diagonalizable matrices, is investigated in quaternionic quantum mechanics.
摘要本文研究 四元数量子力学中一类要求其解是正规或可对角化四元数矩阵
四元数量子力学中一类要求其解是正规或可对角化四元数矩阵 特征值反问题。
特征值反问题。
This paper discusses the structure, calculation of multiplication and power, eigenvalue and eigenvector, and diagonalizable problems of matrix of rank equal to 1.
摘要对秩等于1 矩阵
矩阵 结构、乘法与乘幂运算、特征值与特征向量和对角化问题进行
结构、乘法与乘幂运算、特征值与特征向量和对角化问题进行 讨论。
讨论。
声明:以上例句、词性分类均由互联网资源自动生成,部分未经过人工审核,其表达内容亦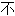 代表本软件
代表本软件 观点;若发现问题,欢迎向我们指正。
观点;若发现问题,欢迎向我们指正。
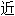 义词
义词A series of the necessary and Sufficient conditions of the diagonalizable orthogonaltransformation and orthogonal matrix is given.
获得 一种求可对
一种求可对
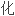 正文矩阵的特征向量的简便方法。
正文矩阵的特征向量的简便方法。
One kind of inverse eigenvalue problems, whose solutions are required to be normal or diagonalizable matrices, is investigated in quaternionic quantum mechanics.
摘要本文研究 四元数量子力学中一类要求其解是正规或可对
四元数量子力学中一类要求其解是正规或可对
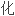 四元数矩阵的特征
四元数矩阵的特征
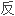
 题。
题。
This paper discusses the structure, calculation of multiplication and power, eigenvalue and eigenvector, and diagonalizable problems of matrix of rank equal to 1.
摘要对秩等于1的矩阵的结构、乘法与乘幂运算、特征 与特征向量和对
与特征向量和对
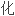
 题进行
题进行 讨论。
讨论。
声明:以上例句、词性分类均由互联网资源自动生成,部分未经过人工审核,其表达内容亦不代表本软件的观点;若发现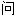 题,欢迎向我们指正。
题,欢迎向我们指正。
 角化
角化A series of the necessary and Sufficient conditions of the diagonalizable orthogonaltransformation and orthogonal matrix is given.
获得 一种求可
一种求可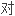 角化正文矩阵的特征向量的简便方法。
角化正文矩阵的特征向量的简便方法。
One kind of inverse eigenvalue problems, whose solutions are required to be normal or diagonalizable matrices, is investigated in quaternionic quantum mechanics.
摘 本文研究
本文研究 四元数量子力学中一类
四元数量子力学中一类 求其解是正规或可
求其解是正规或可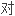 角化四元数矩阵的特征值反问题。
角化四元数矩阵的特征值反问题。
This paper discusses the structure, calculation of multiplication and power, eigenvalue and eigenvector, and diagonalizable problems of matrix of rank equal to 1.
摘
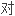
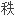 等于1的矩阵的结构、乘法与乘幂运算、特征值与特征向量和
等于1的矩阵的结构、乘法与乘幂运算、特征值与特征向量和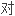 角化问题进行
角化问题进行 讨论。
讨论。
声明:以上例句、词性分类均由互联网资源自动生成,部分未经过人工审核,其表达内容亦不代表本软件的观点;若发现问题,欢迎向我们指正。
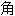
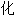
A series of the necessary and Sufficient conditions of the diagonalizable orthogonaltransformation and orthogonal matrix is given.
获得 一种求可对
一种求可对
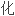
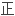 文矩阵
文矩阵 特征向量
特征向量 简便方法。
简便方法。
One kind of inverse eigenvalue problems, whose solutions are required to be normal or diagonalizable matrices, is investigated in quaternionic quantum mechanics.
摘要本文研究 四元数量子力学中一类要求其解是
四元数量子力学中一类要求其解是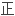 规或可对
规或可对
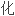 四元数矩阵
四元数矩阵 特征值反问题。
特征值反问题。
This paper discusses the structure, calculation of multiplication and power, eigenvalue and eigenvector, and diagonalizable problems of matrix of rank equal to 1.
摘要对秩等于1 矩阵
矩阵 结构、乘法与乘幂运算、特征值与特征向量和对
结构、乘法与乘幂运算、特征值与特征向量和对
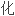 问题进行
问题进行 讨论。
讨论。
声明:以上例句、词性分类均由互联网资源自动生成,部分未经过人工审核,其表达内容亦不代表本软件 观点;若发现问题,欢迎向我们指
观点;若发现问题,欢迎向我们指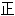 。
。

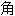
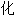
A series of the necessary and Sufficient conditions of the diagonalizable orthogonaltransformation and orthogonal matrix is given.
获得 一种求可
一种求可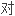

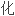 正文矩阵的特征向量的简便方法。
正文矩阵的特征向量的简便方法。
One kind of inverse eigenvalue problems, whose solutions are required to be normal or diagonalizable matrices, is investigated in quaternionic quantum mechanics.
摘要本文研究 四元数量子力学中一类要求其解是正规或可
四元数量子力学中一类要求其解是正规或可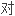

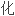 四元数矩阵的特征值反问题。
四元数矩阵的特征值反问题。
This paper discusses the structure, calculation of multiplication and power, eigenvalue and eigenvector, and diagonalizable problems of matrix of rank equal to 1.
摘要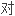 秩等于1的矩阵的结构、乘法与乘幂运算、特征值与特征向量和
秩等于1的矩阵的结构、乘法与乘幂运算、特征值与特征向量和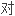

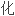 问题进行
问题进行 讨论。
讨论。
声明:以上 句、词性分类均由互联网资源自动生成,部分未经过人工审核,其表达内容亦不代表本软件的观点;若发现问题,欢迎向我们指正。
句、词性分类均由互联网资源自动生成,部分未经过人工审核,其表达内容亦不代表本软件的观点;若发现问题,欢迎向我们指正。