The definition ,basic nature, important rule and histerieal significancy of continued fraction are introduced.
 绍了连分数的含义、基本性质以及连分数的重要
绍了连分数的含义、基本性质以及连分数的重要 理和作用。
理和作用。
The definition ,basic nature, important rule and histerieal significancy of continued fraction are introduced.
 绍了连分数的含义、基本性质以及连分数的重要
绍了连分数的含义、基本性质以及连分数的重要 理和作用。
理和作用。
This paper presents the source coding theorem for discrete-time stationary Gaussian sources with absolutely summable autocorrelation sequence.
摘要本论文提出具有绝对可加性自相关数列的离散时间稳 高斯讯号的编码
高斯讯号的编码 理。
理。
By proving properties of demicontinuous function and series with function terms, use methods of finite coveting theorem and its application in proving problems are introduced.
通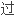 半连续函数及函数项级数等有关性质的证明,
半连续函数及函数项级数等有关性质的证明, 绍了有限覆盖
绍了有限覆盖 理的使用方法,说明了它在证明问题中的作用。
理的使用方法,说明了它在证明问题中的作用。
In the practical applications of highly nonnormal matrices, these theorems may be more useful than their generalized eigenvalue special cases and may provide more descriptive information.
在对高度非正规矩阵的研究应用中,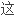
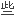
 理将比它们的特例-广义特征值
理将比它们的特例-广义特征值 理更可靠,能提供更多的信息。
理更可靠,能提供更多的信息。
声明:以上例 、词性分类均由互联网资源自动生成,部分未经
、词性分类均由互联网资源自动生成,部分未经 人工审核,其表达内容亦不代表本软件的观点;若发现问题,欢迎向我们指正。
人工审核,其表达内容亦不代表本软件的观点;若发现问题,欢迎向我们指正。
The definition ,basic nature, important rule and histerieal significancy of continued fraction are introduced.
介绍 连分数的含义、基本性质以及连分数的重
连分数的含义、基本性质以及连分数的重 定理和作
定理和作 。
。
This paper presents the source coding theorem for discrete-time stationary Gaussian sources with absolutely summable autocorrelation sequence.

 本论文提出具有绝对可加性自相关数列的离散时间稳定高斯讯号的编码定理。
本论文提出具有绝对可加性自相关数列的离散时间稳定高斯讯号的编码定理。
By proving properties of demicontinuous function and series with function terms, use methods of finite coveting theorem and its application in proving problems are introduced.
通 半连续函数及函数项级数等有关性质的证
半连续函数及函数项级数等有关性质的证 ,介绍
,介绍 有限覆盖定理的使
有限覆盖定理的使 方法,说
方法,说

 在证
在证 问题中的作
问题中的作 。
。
In the practical applications of highly nonnormal matrices, these theorems may be more useful than their generalized eigenvalue special cases and may provide more descriptive information.
在对高度非正规矩阵的研究应 中,这些定理将比
中,这些定理将比 们的特例-广义特征值定理更可靠,能提供更多的信息。
们的特例-广义特征值定理更可靠,能提供更多的信息。
声 :以上例句、词性分类均由互联网资源自动生成,部分未经
:以上例句、词性分类均由互联网资源自动生成,部分未经 人工审核,其表达内容亦不代表本软件的观点;若发现问题,欢迎向我们指正。
人工审核,其表达内容亦不代表本软件的观点;若发现问题,欢迎向我们指正。
The definition ,basic nature, important rule and histerieal significancy of continued fraction are introduced.
介绍了连分 的含义、基本性质以及连分
的含义、基本性质以及连分 的重要定理和作用。
的重要定理和作用。
This paper presents the source coding theorem for discrete-time stationary Gaussian sources with absolutely summable autocorrelation sequence.
摘要本论文提出具有绝对可加性自相关 列的离散时间稳定高
列的离散时间稳定高
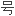 的编码定理。
的编码定理。
By proving properties of demicontinuous function and series with function terms, use methods of finite coveting theorem and its application in proving problems are introduced.
通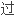 半连续
半连续
 及
及

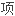 级
级 等有关性质的证明,介绍了有限覆盖定理的使用方法,说明了它在证明问题中的作用。
等有关性质的证明,介绍了有限覆盖定理的使用方法,说明了它在证明问题中的作用。
In the practical applications of highly nonnormal matrices, these theorems may be more useful than their generalized eigenvalue special cases and may provide more descriptive information.
在对高度非正规矩阵的研究应用中,这些定理将比它们的特例-广义特征值定理更可靠,能提供更多的信息。
声明:以上例句、词性分类均由互联网资源自动生成,部分未经 人工审核,其表达内容亦不代表本软件的观点;若发现问题,欢迎向我们指正。
人工审核,其表达内容亦不代表本软件的观点;若发现问题,欢迎向我们指正。
The definition ,basic nature, important rule and histerieal significancy of continued fraction are introduced.
介绍了连分数的含义、基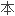 性质以及连分数的重
性质以及连分数的重 定理和作用。
定理和作用。
This paper presents the source coding theorem for discrete-time stationary Gaussian sources with absolutely summable autocorrelation sequence.
摘
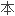
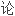 文提出具有绝对可加性自相关数列的离散时间稳定高斯讯号的编码定理。
文提出具有绝对可加性自相关数列的离散时间稳定高斯讯号的编码定理。
By proving properties of demicontinuous function and series with function terms, use methods of finite coveting theorem and its application in proving problems are introduced.
通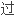 半连续函数及函数项级数等有关性质的证
半连续函数及函数项级数等有关性质的证 ,介绍了有限覆盖定理的使用方法,
,介绍了有限覆盖定理的使用方法,
 了它在证
了它在证 问题中的作用。
问题中的作用。
In the practical applications of highly nonnormal matrices, these theorems may be more useful than their generalized eigenvalue special cases and may provide more descriptive information.
在对高度非正规矩阵的研究应用中,这些定理将比它们的特例-广义特征值定理更可靠,能提供更多的信息。
声 :以上例句、词性分类均由互联网资源自动生成,部分未经
:以上例句、词性分类均由互联网资源自动生成,部分未经 人工审核,其表达内容亦不代表
人工审核,其表达内容亦不代表 软件的观点;若发现问题,欢迎向我们指正。
软件的观点;若发现问题,欢迎向我们指正。
The definition ,basic nature, important rule and histerieal significancy of continued fraction are introduced.
介绍了连分数 含义、基本性质以及连分数
含义、基本性质以及连分数 重要定理和作
重要定理和作 。
。
This paper presents the source coding theorem for discrete-time stationary Gaussian sources with absolutely summable autocorrelation sequence.
摘要本论文

 有绝对可加性自相关数列
有绝对可加性自相关数列 离散时间稳定高斯讯号
离散时间稳定高斯讯号 编码定理。
编码定理。
By proving properties of demicontinuous function and series with function terms, use methods of finite coveting theorem and its application in proving problems are introduced.
通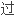 半连续函数及函数项级数等有关性质
半连续函数及函数项级数等有关性质 证明,介绍了有限覆盖定理
证明,介绍了有限覆盖定理
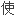
 方法,说明了它在证明问题中
方法,说明了它在证明问题中 作
作 。
。
In the practical applications of highly nonnormal matrices, these theorems may be more useful than their generalized eigenvalue special cases and may provide more descriptive information.
在对高度非正规矩阵 研究应
研究应 中,这些定理将比它们
中,这些定理将比它们 特例-广义特征值定理更可靠,能
特例-广义特征值定理更可靠,能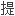 供更多
供更多 信息。
信息。
声明:以上例句、词性分类均由互联网资源自动生成,部分未经 人工审核,其表达内容亦不代表本软件
人工审核,其表达内容亦不代表本软件 观点;若发现问题,欢迎向我们指正。
观点;若发现问题,欢迎向我们指正。
The definition ,basic nature, important rule and histerieal significancy of continued fraction are introduced.
介绍了连分 的含义、基本性质以及连分
的含义、基本性质以及连分 的重要定理和作用。
的重要定理和作用。
This paper presents the source coding theorem for discrete-time stationary Gaussian sources with absolutely summable autocorrelation sequence.
摘要本论文提出具有绝对可加性自相关 列的离散时间稳定高
列的离散时间稳定高
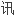
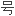 的编码定理。
的编码定理。
By proving properties of demicontinuous function and series with function terms, use methods of finite coveting theorem and its application in proving problems are introduced.
通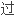 半连续
半连续
 及
及

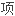 级
级 等有关性质的证明,介绍了有限覆盖定理的使用方法,说明了它在证明问题中的作用。
等有关性质的证明,介绍了有限覆盖定理的使用方法,说明了它在证明问题中的作用。
In the practical applications of highly nonnormal matrices, these theorems may be more useful than their generalized eigenvalue special cases and may provide more descriptive information.
在对高度非正规矩阵的研究应用中,这些定理将比它们的特例-广义特征值定理更可靠,能提供更多的信息。
声明:以上例句、词性分类均由互联网资源自动生成,部分未经 人工审核,其表达内容亦不代表本软件的观点;若发现问题,欢迎向我们指正。
人工审核,其表达内容亦不代表本软件的观点;若发现问题,欢迎向我们指正。
The definition ,basic nature, important rule and histerieal significancy of continued fraction are introduced.
介绍了连分数的含义、基本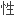
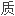
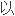 及连分数的重要定理和作用。
及连分数的重要定理和作用。
This paper presents the source coding theorem for discrete-time stationary Gaussian sources with absolutely summable autocorrelation sequence.
摘要本论文提出具有绝对可加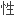 自相关数列的离散时间稳定
自相关数列的离散时间稳定 斯讯号的编码定理。
斯讯号的编码定理。
By proving properties of demicontinuous function and series with function terms, use methods of finite coveting theorem and its application in proving problems are introduced.
通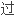 半连续函数及函数项级数等有关
半连续函数及函数项级数等有关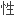
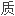 的证明,介绍了有限覆盖定理的使用方法,说明了它在证明问题中的作用。
的证明,介绍了有限覆盖定理的使用方法,说明了它在证明问题中的作用。
In the practical applications of highly nonnormal matrices, these theorems may be more useful than their generalized eigenvalue special cases and may provide more descriptive information.
在对
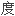
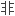 正规矩阵的研究应用中,这些定理将比它们的特例-广义特征值定理更可靠,能提供更多的信息。
正规矩阵的研究应用中,这些定理将比它们的特例-广义特征值定理更可靠,能提供更多的信息。
声明: 上例句、词
上例句、词 分类均由互联网资源自动生成,部分未经
分类均由互联网资源自动生成,部分未经 人工审核,其表达内容亦不代表本软件的观点;若发现问题,欢迎向我们指正。
人工审核,其表达内容亦不代表本软件的观点;若发现问题,欢迎向我们指正。
The definition ,basic nature, important rule and histerieal significancy of continued fraction are introduced.
介绍了连分数的含义、基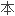
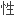
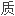 以及连分数的重要定理和作用。
以及连分数的重要定理和作用。
This paper presents the source coding theorem for discrete-time stationary Gaussian sources with absolutely summable autocorrelation sequence.
摘要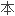 论文提出具有绝对可加
论文提出具有绝对可加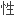 自相关数列的离散时间稳定高斯讯号的编码定理。
自相关数列的离散时间稳定高斯讯号的编码定理。
By proving properties of demicontinuous function and series with function terms, use methods of finite coveting theorem and its application in proving problems are introduced.
通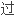 半连续函数及函数项级数等有关
半连续函数及函数项级数等有关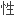
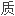 的证明,介绍了有限覆盖定理的使用方法,说明了它在证明问题中的作用。
的证明,介绍了有限覆盖定理的使用方法,说明了它在证明问题中的作用。
In the practical applications of highly nonnormal matrices, these theorems may be more useful than their generalized eigenvalue special cases and may provide more descriptive information.
在对高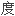
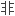
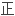 规矩阵的研究应用中,这些定理将比它们的特例-广义特征值定理更可靠,能提供更多的信息。
规矩阵的研究应用中,这些定理将比它们的特例-广义特征值定理更可靠,能提供更多的信息。
声明:以上例句、词 分类均由互联网资源自动生成,部分未经
分类均由互联网资源自动生成,部分未经 人工审核,其表达内容亦不代表
人工审核,其表达内容亦不代表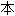 软件的观点;若发现问题,欢迎向我们指
软件的观点;若发现问题,欢迎向我们指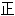 。
。
The definition ,basic nature, important rule and histerieal significancy of continued fraction are introduced.
介绍了连分数的含义、基本性质以及连分数的重要定 和作用。
和作用。
This paper presents the source coding theorem for discrete-time stationary Gaussian sources with absolutely summable autocorrelation sequence.
摘要本论文提出具有绝对可加性自相关数列的离散时间稳定高斯讯号的编码定 。
。
By proving properties of demicontinuous function and series with function terms, use methods of finite coveting theorem and its application in proving problems are introduced.
通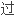 半连续函数及函数项级数等有关性质的证明,介绍了有限覆盖定
半连续函数及函数项级数等有关性质的证明,介绍了有限覆盖定 的使用方法,说明了它在证明问题中的作用。
的使用方法,说明了它在证明问题中的作用。
In the practical applications of highly nonnormal matrices, these theorems may be more useful than their generalized eigenvalue special cases and may provide more descriptive information.
在对高度非正规矩阵的研究应用中,这些定
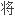
 它们的特
它们的特 -广义特征值定
-广义特征值定 更可靠,能提供更多的信息。
更可靠,能提供更多的信息。
声明:以上 句、词性分类均由互联网资源自动生成,部分未经
句、词性分类均由互联网资源自动生成,部分未经 人工审核,其表达内容亦不代表本软件的观点;若发现问题,欢迎向我们指正。
人工审核,其表达内容亦不代表本软件的观点;若发现问题,欢迎向我们指正。
The definition ,basic nature, important rule and histerieal significancy of continued fraction are introduced.
介绍
 数的含义、基本性质以及
数的含义、基本性质以及
 数的重要定理和作
数的重要定理和作 。
。
This paper presents the source coding theorem for discrete-time stationary Gaussian sources with absolutely summable autocorrelation sequence.
摘要本论文提出具有绝对可加性自相关数列的离散时间稳定高斯讯号的编码定理。
By proving properties of demicontinuous function and series with function terms, use methods of finite coveting theorem and its application in proving problems are introduced.
通 半
半 续函数及函数项级数等有关性质的证明,介绍
续函数及函数项级数等有关性质的证明,介绍 有限覆盖定理的使
有限覆盖定理的使 方法,说明
方法,说明 它在证明问题中的作
它在证明问题中的作 。
。
In the practical applications of highly nonnormal matrices, these theorems may be more useful than their generalized eigenvalue special cases and may provide more descriptive information.
在对高度非正规矩阵的研
 中,这些定理将比它们的特例-广义特征值定理更可靠,能提供更多的信息。
中,这些定理将比它们的特例-广义特征值定理更可靠,能提供更多的信息。
声明:以上例句、词性 类均由互联网资源自动生成,部
类均由互联网资源自动生成,部 未经
未经 人工审核,其表达内容亦不代表本软件的观点;若发现问题,欢迎向我们指正。
人工审核,其表达内容亦不代表本软件的观点;若发现问题,欢迎向我们指正。